Water tank problem

I solved this problem using ratios and proportions, also using references to the dimensions of a real bike. From the picture of the water tank, I can see that the bike is leaning on the soup can, so using the height of the bike should be a good estimate. I see that from the ground (or the right edge of the soup can) to the letter “O”, it is the height from ground to the back rack of the bike. 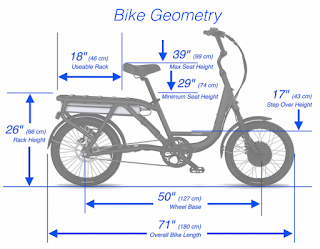And from the ground (or the right edge of the soup can) to the letter “P”, it is the height from the ground to the seat height. I found a picture of the dimensions of the bikes online and used this photo as a reference. The height of the back rack is 66cm and the height of the seat is 74 cm.

Using a ruler, from the actual picture of the soup can I measured the diameter of the can (from the left edge to the right edge), which is around 3.4cm. From the left edge to the letter “T” is 0.5 cm and same with the right edge to the letter “0”. I also measured from the right edge to the letter “P”, which is around 0.7 cm. I found the ratio of the actual picture to the bike rack which is 0.5cm/66cm = 0.007575756. And the ratio of the actual picture to the seat is 0.7cm/ 74cm= 0.00946. Due to different adjustments to the seat positions and different dimension of bikes, I am going to take the average ratio of soup can to bike as 0.0085. And since the diameter of the picture 3.4 cm, the diameter of the water tank should be 3.4/0.0085= 400cm. The height of the soup can picture is 5cm, so using the same ratio, the height of the water tank should be around 5 cm/0.0085 cm=  588.24cm.

picture from the internet:http://static1.1.sqspcdn.com/static/f/331681/25655817/1415671280363/JR_U500V3+Geometry+graphicweb.gif?token=DAJnpFQynD0URdPDXwg9IuBwzCw%3D